ローレンツ方程式

ローレンツ方程式(ローレンツほうていしき)とは、数学者・気象学者であるエドワード・ローレンツ(Edward Lorenz)が最初に研究した非線型常微分方程式である。特定のパラメータ値と初期条件に対してカオス的な解を持つことで注目されている。特に、ローレンツ方程式のカオス解の集合はローレンツ・アトラクターと呼ばれる。いわゆるバタフライ効果の説明に用いられることが多く、決定論的な連立常微分方程式が初期値鋭敏性を持つことは驚きをもって迎えられ、カオス研究の端緒となった。
概要
[編集]1963年、エドワード・ローレンツは、数値シミュレーションや数値計算を担当したエレン・フェッターと、ローレンツ方程式の発見に至る初期の数値計算を担当したソフトウェアエンジニア、マーガレット・ハミルトンの協力を得て、大気変動の簡易数学モデルを開発した[1][2]。このモデルが、現在ローレンツ方程式として知られている以下の3つの常微分方程式の系である:
この方程式は、下から暖められ、上から冷やされる2次元の流体層の特性に関するもので、3つの量の時間に対する変化率を記述しており、 xは対流速度に、yは水平温度変化に、zは垂直温度変化に比例する。 [3] また定数 σ, ρ, β はそれぞれ プラントル数、レイリー数に関する不安定度を表すパラメータ、臨界水平波数に関するパラメータである。
ローレンツ方程式はレーザー、[4] 発電機、[5]サーモサイフォン、 [6] ブラシレスDCモーター、 [7] 電気回路、 [8] 化学反応[9]、正浸透 [10]などの簡易モデルで生じうる。また、マルクス水車のフーリエ空間での支配方程式でもある [11][12]。すなわちマルクス水車はカオス運動を示し、一定速度で一方向に回転するのではなく、その回転が加速したり減速したり停止したり方向転換したり、それらの組み合わせで前後に振動したりと予測不能の動きをする。
解析
[編集]通常、パラメータ σ, ρ, β は正であると仮定する。ローレンツはσ=10、β=8/3、ρ=28という値を使用し、これらの値(およびその近傍の値)に対して、系がカオス的な振る舞いをすることを示している[13]。
もしρ<1なら、均衡点は1つだけであり、それは原点である。この点は対流がないことに対応する。すべての軌道は原点に収束し、広域的なアトラクターとなる。[14].
ρ = 1でピッチフォーク分岐が起こり、ρ > 1 でさらに下記の2つの臨界点が現れる。 これらは定常対流に相当する。この二つの平衡点は の場合にのみ安定である。これはσ > β + 1の時のみ、 ρは正となりうる。また臨界値では、両平衡点はホップ分岐を経て安定性を失う。[15]
ρ=28、σ=10、β=8/3のとき、ローレンツ方程式はカオス解を持つ(ただし全ての解がカオスであるとは言えない)。ほぼ全ての初期点は、3つの平衡に関して、不変集合 – ローレンツアトラクター – 、ストレンジアトラクター、フラクタル、自己励起アトラクタに傾くことになる。そのハウスドルフ次元は、上からリアプノフ指数 によって2.06±0.01と見積もられる[16] 。また相関次元は2.05±0.01と推定されている[17]。 グローバルアトラクターの正確なリアプノフ指数の公式は、パラメータの古典的な制限の下で解析的に求めることができ、次に示す[18][16][19]。
ローレンツアトラクターは解析が難しいが、微分方程式のアトラクターへの作用はかなり単純な幾何学モデルで記述され[20]、この証明はスメイルの問題の14番目の問題であったが、2002年にウォリック・タッカーによって初めて解決された[21]。
ρの他の値では、系は結び目のある周期的な軌道を示す。例えば、ρ=99.96ではT(3,2)(トーラス結び目)となる。
| 異なるρに対するローレンツ方程式の解の例 | |
|---|---|

|

|
| ρ = 14, σ = 10, β = 8/3 (拡大) | ρ = 13, σ = 10, β = 8/3 (拡大) |
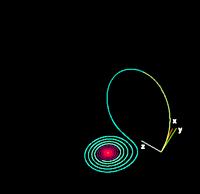
|

|
| ρ = 15, σ = 10, β = 8/3 (拡大) | ρ = 28, σ = 10, β = 8/3 (拡大) |
| 小さいρでは系は安定し、2つの固定点のうち、いずれかの点アトラクターに進展する。ρ > 24.74では, 固定点は斥力源となり、軌道はそれらに反発して非常に複雑な形となる。 | |
| 初期値に対する鋭敏性 | ||
|---|---|---|
| Time t = 1 (拡大) | Time t = 2 (拡大) | Time t = 3 (拡大) |

|

|

|
| ρ = 28, σ = 10, β = 8/3の条件で生成されたこれらの画像は二つの軌跡(青と黄色)の時間発展を示している。二つの軌跡の初期値はx座標のみ10−5の差がつけられている。初め、二つの軌跡は一致しているように見える(青色の上から黄色が描かれているため黄色の軌跡だけ見える)が、時間経過と共に明らかに分岐していくのがわかる。 | ||
テント写像との関連
[編集]
ローレンツの論文[2]の図4において、 ローレンツは、系が到達したz方向の相対最大値を、z方向のそれより以前の相対最大値に対してプロットした。この手順は後にローレンツマップとして知られるようになった(軌跡と所定の曲面の交点をプロットするポアンカレプロットと混同しないように)。結果としてこのプロットはテント写像に非常によく似た形をしており、ローレンツは、z の最大値があるカットオフ値を超えると、系が片方のローブ(軌跡上の片方の円盤)に切り替わることを発見した。これをテント写像で知られているカオスと組み合わせることで、系が2つのローブの間をカオス的に行き来することが判明した。
一般化されたローレンツ方程式
[編集]ローレンツ方程式の発見後高次元のローレンツモデルに関する論文が相次ぎ、一般化されたローレンツモデルが作成された[22]。このモデルは、3つの状態変数に対する古典的なローレンツモデル、または5つの状態変数に対する以下の5次元ローレンツモデルに単純化することができる[23]。
他のパラメータの値によらず、d0 = 19/3である[22][23]。
シミュレーション
[編集]MATLAB
[編集]% Solve over time interval [0,100] with initial conditions [1,1,1]
% ''f'' is set of differential equations
% ''a'' is array containing x, y, and z variables
% ''t'' is time variable
sigma = 10;
beta = 8/3;
rho = 28;
f = @(t,a) [-sigma*a(1) + sigma*a(2); rho*a(1) - a(2) - a(1)*a(3); -beta*a(3) + a(1)*a(2)];
[t,a] = ode45(f,[0 100],[1 1 1]); % Runge-Kutta 4th/5th order ODE solver
plot3(a(:,1),a(:,2),a(:,3))
Mathematica
[編集]標準的な記法:
tend = 50;
eq = {x'[t] == σ (y[t] - x[t]),
y'[t] == x[t] (ρ - z[t]) - y[t],
z'[t] == x[t] y[t] - β z[t]};
init = {x[0] == 10, y[0] == 10, z[0] == 10};
pars = {σ->10, ρ->28, β->8/3};
{xs, ys, zs} =
NDSolveValue[{eq /. pars, init}, {x, y, z}, {t, 0, tend}];
ParametricPlot3D[{xs[t], ys[t], zs[t]}, {t, 0, tend}]
冗長性を抑えたバージョン:
lorenz = NonlinearStateSpaceModel[{{σ (y - x), x (ρ - z) - y, x y - β z}, {}}, {x, y, z}, {σ, ρ, β}];
soln[t_] = StateResponse[{lorenz, {10, 10, 10}}, {10, 28, 8/3}, {t, 0, 50}];
ParametricPlot3D[soln[t], {t, 0, 50}]
応用
[編集]大気の対流モデル
[編集]原論文で言及される通り[24]、ローレンツ系はバリー・サルツマンが以前に研究したより大きな系を縮小したものであり[25]、ローレンツ方程式は下から一様に加熱され、上から一様に冷却される浅い流体層における流体循環を記述する方程式をブシネスク近似から導いたものである。[24] この流体循環はレイリー・ベナール対流と呼ばれる。流体は2次元(垂直と水平)に循環すると仮定し、矩形の周期的境界条件を設定する。[24]
系の流れ関数と温度をモデル化したこの偏微分方程式を,スペクトルガラーキン近似を用いて,流体力学場をフーリエ級数で展開し,流れ関数については1次,温度については2次で切り捨てる。これにより、方程式は3つの連立した非線形常微分方程式に縮小される。詳細な導出は, Hilborn (2000)による非線形力学の教科書、Appendix C; Bergé, Pomeau & Vidal (1984), Appendix D、または Shen (2016)[26]を参照。
大気中のカオスと秩序の性質を示すモデル
[編集]低次元ローレンツモデルに見られるカオス的な特徴が、地球大気の特徴(気象のカオス性)を表しうることが認められている[27][28][29]。一方で、一般化されたローレンツモデルと初期のローレンツモデルにおいてカオスと予測可能な振る舞いが共存していることから[22][30][31]、Shenとその共著者[29][32]は「気象はカオスと、明確な予測可能性を持つ秩序の両方を持っている」という改訂見解を提案した。従来の見解を発展させたこの見解は、「理論的なローレンツモデルに見られるカオスと規則的な特徴は、地球の大気の特徴をよりよく表しうる」と示唆するために用いられている。
スメイルの14番目の問題
[編集]スメイルの14番目の問題は「ローレンツアトラクターはストレンジアトラクターの性質を持つか?」というものであった。この問題は2002年にウォリック・タッカーによって肯定的に解決された[21]。証明には 区間演算、正準系などの厳密な数値計算が用いられた。初めにタッカーは流れの軌跡によって横方向に切断したものである断面積を定義した。ここから、各に対して の軌跡が初めてに交わる点とするfirst-return写像 を定義できる。
続く証明は3つのポイントに分かれてなされ、ストレンジアトラクターの存在を示唆する[33]。
- the first-return写像で不変、すなわちなる区域が存在する。
- The return写像はforward invariant cone fieldを認める
- この不変円錐場内のベクトルは、return写像の微分によって一様に拡大される。
第一段階では断面積がによって二つの弧に分割される事実を用いる[33]。タッカーはこの二つの弧を小さな長方形で覆い、これらの長方形の集合がを与えることを考えた。このことを証明するためには、の全ての点がの中のに戻ってくるのをみれば良い。そのために、の下方にという小さな距離でを想定し、の中心とオイラーの積分法を用いて、点からの流れが内に与える新たな点を計算できる。そうしてテイラー展開によって内の点が内のどこに写されるかを知ることができる。これによって中心がで与えられる新たな長方形が得られる。したがって内のすべての点はの中に写される。あとは軌跡の流れが内に戻ってくるまでこの方法を再帰的に実行し、となるに入る長方形を得れば良いのであるが、問題はこの工程を何度か繰り返すうちに推定が不正確になることである。そこでタッカーはをより小さい長方形に分割し、処理を再帰的に適応した。もう一つの問題は、このアルゴリズムを適用しているうちに、流れがより「水平」になってしまい[33]、不正確さが飛躍的に増大することで、これを防ぐためにはアルゴリズムでは断面の向きを変え、水平または垂直になるようにする必要があった。
ギャラリー
[編集]-
A solution in the xz平面で描写された高解像度のローレンツアトラクター
-
SVGでレンダリングされたローレンツアトラクター
-
ローレンツ方程式での複数の軌跡のアニメーション
-
金属製ワイヤーで製作されたローレンツアトラクターの3Dモデル
-
ローレンツ系の近傍解の発散を示すアニメーション
-
断続的なサイクルにあるローレンツアトラクターを可視化したもの
-
ローレンツ系における2つの流線(ρ = 0 から ρ = 28, σ = 10, β = 8/3)
-
ローレンツ系のρ依存性のアニメーション
-
Brain Dynamics Toolboxのローレンツアトラクターのアニメーション[34]
関連項目
[編集]- バタフライ効果
- カオス理論
- ロジスティック方程式
- レスラー方程式
- 常微分方程式
- Eden's conjecture on the Lyapunov dimension
- Lorenz 96 model
- List of chaotic maps
- Takens' theorem
脚注
[編集]- ^ Lorenz (1960)
- ^ a b Lorenz (1963)
- ^ Sparrow (1982)
- ^ Haken (1975)
- ^ Knobloch (1981)
- ^ Gorman, Widmann & Robbins (1986)
- ^ Hemati (1994)
- ^ Cuomo & Oppenheim (1993)
- ^ Poland (1993)
- ^ Tzenov (2014)[要出典]
- ^ Kolář & Gumbs (1992)
- ^ Mishra & Sanghi (2006)
- ^ Hirsch, Smale & Devaney (2003), pp.303-305
- ^ Hirsch, Smale & Devaney (2003), pp.306+307
- ^ Hirsch, Smale & Devaney (2003), pp. 307–308
- ^ a b Kuznetsov, N.V.; Mokaev, T.N.; Kuznetsova, O.A.; Kudryashova, E.V. (2020). “The Lorenz system: hidden boundary of practical stability and the Lyapunov dimension”. Nonlinear Dynamics 102 (2): 713–732. doi:10.1007/s11071-020-05856-4.
- ^ Grassberger & Procaccia (1983)
- ^ Leonov et al. (2016)
- ^ Kuznetsov, Nikolay; Reitmann, Volker (2021). Attractor Dimension Estimates for Dynamical Systems: Theory and Computation. Cham: Springer
- ^ Guckenheimer, John; Williams, R. F. (1979-12-01). “Structural stability of Lorenz attractors”. Publications Mathématiques de l'Institut des Hautes Études Scientifiques 50 (1): 59–72. doi:10.1007/BF02684769. ISSN 0073-8301.
- ^ a b Tucker (2002)
- ^ a b c Shen, Bo-Wen (2019-03-01). “Aggregated Negative Feedback in a Generalized Lorenz Model”. International Journal of Bifurcation and Chaos 29 (3): 1950037–1950091. Bibcode: 2019IJBC...2950037S. doi:10.1142/S0218127419500378. ISSN 0218-1274.
- ^ a b Shen, Bo-Wen (2014-04-28). “Nonlinear Feedback in a Five-Dimensional Lorenz Model”. Journal of the Atmospheric Sciences 71 (5): 1701–1723. Bibcode: 2014JAtS...71.1701S. doi:10.1175/jas-d-13-0223.1. ISSN 0022-4928.
- ^ a b c Lorenz (1963)
- ^ Saltzman (1962)
- ^ Shen, B.-W. (2015-12-21). “Nonlinear feedback in a six-dimensional Lorenz model: impact of an additional heating term” (英語). Nonlinear Processes in Geophysics 22 (6): 749–764. Bibcode: 2015NPGeo..22..749S. doi:10.5194/npg-22-749-2015. ISSN 1607-7946.
- ^ Ghil, Michael; Read, Peter; Smith, Leonard (2010-07-23). “Geophysical flows as dynamical systems: the influence of Hide's experiments”. Astronomy & Geophysics 51 (4): 4.28–4.35. Bibcode: 2010A&G....51d..28G. doi:10.1111/j.1468-4004.2010.51428.x. ISSN 1366-8781.
- ^ Read, P. (1993). Application of Chaos to Meteorology and Climate. In The Nature of Chaos; Mullin, T., Ed. Oxford, UK: Oxford Science Publications. pp. 220–260. ISBN 0198539541
- ^ a b Shen, Bo-Wen; Pielke, Roger; Zeng, Xubin; Cui, Jialin; Faghih-Naini, Sara; Paxson, Wei; Kesarkar, Amit; Zeng, Xiping et al. (2022-11-12). “The Dual Nature of Chaos and Order in the Atmosphere” (英語). Atmosphere 13 (11): 1892. Bibcode: 2022Atmos..13.1892S. doi:10.3390/atmos13111892. ISSN 2073-4433.
- ^ Yorke, James A.; Yorke, Ellen D. (1979-09-01). “Metastable chaos: The transition to sustained chaotic behavior in the Lorenz model” (英語). Journal of Statistical Physics 21 (3): 263–277. Bibcode: 1979JSP....21..263Y. doi:10.1007/BF01011469. ISSN 1572-9613.
- ^ Shen, Bo-Wen; Pielke, R. A.; Zeng, X.; Baik, J.-J.; Faghih-Naini, S.; Cui, J.; Atlas, R.; Reyes, T. A. L. (2021), Skiadas, Christos H.; Dimotikalis, Yiannis, eds., “Is Weather Chaotic? Coexisting Chaotic and Non-chaotic Attractors Within Lorenz Models” (英語), 13th Chaotic Modeling and Simulation International Conference (Cham: Springer International Publishing): pp. 805–825, doi:10.1007/978-3-030-70795-8_57, ISBN 978-3-030-70794-1 2022年12月22日閲覧。
- ^ Shen, Bo-Wen; Pielke, Roger A.; Zeng, Xubin; Baik, Jong-Jin; Faghih-Naini, Sara; Cui, Jialin; Atlas, Robert (2021-01-01). “Is Weather Chaotic?: Coexistence of Chaos and Order within a Generalized Lorenz Model” (英語). Bulletin of the American Meteorological Society 102 (1): E148–E158. Bibcode: 2021BAMS..102E.148S. doi:10.1175/BAMS-D-19-0165.1. ISSN 0003-0007.
- ^ a b c Viana (2000)
- ^ Heitmann, S., Breakspear, M (2017-2022) Brain Dynamics Toolbox. bdtoolbox.org doi.org/10.5281/zenodo.5625923
参考文献
[編集]- Bergé, Pierre; Pomeau, Yves; Vidal, Christian (1984). Order within Chaos: Towards a Deterministic Approach to Turbulence. New York: John Wiley & Sons. ISBN 978-0-471-84967-4
- Cuomo, Kevin M.; Oppenheim, Alan V. (1993). “Circuit implementation of synchronized chaos with applications to communications”. Physical Review Letters 71 (1): 65–68. Bibcode: 1993PhRvL..71...65C. doi:10.1103/PhysRevLett.71.65. ISSN 0031-9007. PMID 10054374.
- Gorman, M.; Widmann, P.J.; Robbins, K.A. (1986). “Nonlinear dynamics of a convection loop: A quantitative comparison of experiment with theory”. Physica D 19 (2): 255–267. Bibcode: 1986PhyD...19..255G. doi:10.1016/0167-2789(86)90022-9.
- Grassberger, P.; Procaccia, I. (1983). “Measuring the strangeness of strange attractors”. Physica D 9 (1–2): 189–208. Bibcode: 1983PhyD....9..189G. doi:10.1016/0167-2789(83)90298-1.
- Haken, H. (1975). “Analogy between higher instabilities in fluids and lasers”. Physics Letters A 53 (1): 77–78. Bibcode: 1975PhLA...53...77H. doi:10.1016/0375-9601(75)90353-9.
- Hemati, N. (1994). “Strange attractors in brushless DC motors”. IEEE Transactions on Circuits and Systems I: Fundamental Theory and Applications 41 (1): 40–45. doi:10.1109/81.260218. ISSN 1057-7122.
- Hilborn, Robert C. (2000). Chaos and Nonlinear Dynamics: An Introduction for Scientists and Engineers (second ed.). Oxford University Press. ISBN 978-0-19-850723-9
- Hirsch, Morris W.; Smale, Stephen; Devaney, Robert (2003). Differential Equations, Dynamical Systems, & An Introduction to Chaos (Second ed.). Boston, MA: Academic Press. ISBN 978-0-12-349703-1
- Knobloch, Edgar (1981). “Chaos in the segmented disc dynamo”. Physics Letters A 82 (9): 439–440. Bibcode: 1981PhLA...82..439K. doi:10.1016/0375-9601(81)90274-7.
- Kolář, Miroslav; Gumbs, Godfrey (1992). “Theory for the experimental observation of chaos in a rotating waterwheel”. Physical Review A 45 (2): 626–637. Bibcode: 1992PhRvA..45..626K. doi:10.1103/PhysRevA.45.626. PMID 9907027.
- Leonov, G.A.; Kuznetsov, N.V.; Korzhemanova, N.A.; Kusakin, D.V. (2016). “Lyapunov dimension formula for the global attractor of the Lorenz system”. Communications in Nonlinear Science and Numerical Simulation 41: 84–103. arXiv:1508.07498. Bibcode: 2016CNSNS..41...84L. doi:10.1016/j.cnsns.2016.04.032.
- Lorenz, Edward Norton (1963). “Deterministic nonperiodic flow”. Journal of the Atmospheric Sciences 20 (2): 130–141. Bibcode: 1963JAtS...20..130L. doi:10.1175/1520-0469(1963)020<0130:DNF>2.0.CO;2.
- Mishra, Aashwin; Sanghi, Sanjeev (2006). “A study of the asymmetric Malkus waterwheel: The biased Lorenz equations”. Chaos: An Interdisciplinary Journal of Nonlinear Science 16 (1): 013114. Bibcode: 2006Chaos..16a3114M. doi:10.1063/1.2154792. PMID 16599745.
- Pchelintsev, A.N. (2014). “Numerical and Physical Modeling of the Dynamics of the Lorenz System”. Numerical Analysis and Applications 7 (2): 159–167. doi:10.1134/S1995423914020098.
- Poland, Douglas (1993). “Cooperative catalysis and chemical chaos: a chemical model for the Lorenz equations”. Physica D 65 (1): 86–99. Bibcode: 1993PhyD...65...86P. doi:10.1016/0167-2789(93)90006-M.
- Saltzman, Barry (1962). “Finite Amplitude Free Convection as an Initial Value Problem—I”. Journal of the Atmospheric Sciences 19 (4): 329–341. Bibcode: 1962JAtS...19..329S. doi:10.1175/1520-0469(1962)019<0329:FAFCAA>2.0.CO;2.
- Shen, B.-W. (2015-12-21). "Nonlinear feedback in a six-dimensional Lorenz model: impact of an additional heating term". Nonlinear Processes in Geophysics. 22 (6): 749–764. doi:10.5194/npg-22-749-2015. ISSN 1607-7946.
- Sparrow, Colin (1982). The Lorenz Equations: Bifurcations, Chaos, and Strange Attractors. Springer
- Tucker, Warwick (2002). “A Rigorous ODE Solver and Smale's 14th Problem”. Foundations of Computational Mathematics 2 (1): 53–117. doi:10.1007/s002080010018.
- Tzenov, Stephan (2014). "Strange Attractors Characterizing the Osmotic Instability". arXiv:1406.0979v1 [physics.flu-dyn]。
- Viana, Marcelo (2000). “What's new on Lorenz strange attractors?”. The Mathematical Intelligencer 22 (3): 6–19. doi:10.1007/BF03025276.
- Lorenz, Edward N. (1960). “The statistical prediction of solutions of dynamic equations.”. Symposium on Numerical Weather Prediction in Tokyo.
詳細
[編集]- G.A. Leonov & N.V. Kuznetsov (2015). “On differences and similarities in the analysis of Lorenz, Chen, and Lu systems”. Applied Mathematics and Computation 256: 334–343. doi:10.1016/j.amc.2014.12.132.
- Pchelintsev, A.N. (2022). “On a high-precision method for studying attractors of dynamical systems and systems of explosive type”. Mathematics 10 (8): 1207. arXiv:2206.08195. doi:10.3390/math10081207.
外部リンク
[編集]- Hazewinkel, Michiel, ed. (2001), “Lorenz attractor”, Encyclopaedia of Mathematics, Springer, ISBN 978-1-55608-010-4
- Weisstein, Eric W. "Lorenz attractor". mathworld.wolfram.com (英語).
- Lorenz attractor by Rob Morris, Wolfram Demonstrations Project.
- Lorenz equation on planetmath.org
- Synchronized Chaos and Private Communications, with Kevin Cuomo. The implementation of Lorenz attractor in an electronic circuit.
- Lorenz attractor interactive animation (you need the Adobe Shockwave plugin)
- 3D Attractors: Mac program to visualize and explore the Lorenz attractor in 3 dimensions
- Lorenz Attractor implemented in analog electronic
- Lorenz Attractor interactive animation (implemented in Ada with GTK+. Sources & executable)
- Web based Lorenz Attractor (implemented in JavaScript/HTML/CSS)
- Interactive web based Lorenz Attractor made with Iodide
- ローレンツアトラクタを描画する、あるいはそれに類似することをするときのために (英語版)

![{\displaystyle {\begin{aligned}{\frac {\mathrm {d} x}{\mathrm {d} t}}&=\sigma (y-x),\\[6pt]{\frac {\mathrm {d} y}{\mathrm {d} t}}&=x(\rho -z)-y,\\[6pt]{\frac {\mathrm {d} z}{\mathrm {d} t}}&=xy-\beta z.\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7928004d58943529a7be774575a62ca436a82a7f)



![{\displaystyle {\begin{aligned}{\frac {\mathrm {d} x}{\mathrm {d} t}}&=\sigma (y-x),\\[6pt]{\frac {\mathrm {d} y}{\mathrm {d} t}}&=x(\rho -z)-y,\\[6pt]{\frac {\mathrm {d} z}{\mathrm {d} t}}&=xy-xy_{1}-\beta z,\\[6pt]{\frac {\mathrm {d} y_{1}}{\mathrm {d} t}}&=xz-2xz_{1}-d_{0}y_{1},\\[6pt]{\frac {\mathrm {d} z_{1}}{\mathrm {d} t}}&=2xy_{1}-4\beta z_{1}.\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/34c363e59f18d5689d3af72f5fdbcbc5d7f2abea)


























![Brain Dynamics Toolboxのローレンツアトラクターのアニメーション[34]](http://upload.wikimedia.org/wikipedia/commons/thumb/f/f8/Lorenz_Attractor_Brain_Dynamics_Toolbox.gif/120px-Lorenz_Attractor_Brain_Dynamics_Toolbox.gif)