Template:Infobox mathematical function

テンプレートの解説[表示] [編集] [履歴] [キャッシュを破棄]

name
[[File:{{{image}}}|frameless]] caption
基礎的な情報
考案者	deriver
導入の動機	motivation_of_creation
考案時期	date
拡張元	extends
応用分野	fields_of_application
主な応用先	main_applications
領域
定義域	domain
終域	codomain
値域	range
基本的な特徴
偶奇	parity
周期	period
解析的？	analytic
有理型？	meromorphic
正則？	holomorphic
特筆すべき値
原点での値	zero
+∞での値	plusinf
−∞での値	minusinf
極大値	max
極小値	min
vr1での値	f1
vr2での値	f2
[...]での値	[...]
vr5での値	f5
その他の特徴
漸近線	asymptote
零点	root
臨界点	critical
変曲点	inflection
不動点	fixed
極	poles
関連する関数
逆数	reciprocal
逆関数	inverse
導関数	derivative
原始関数	antiderivative
その他	other_related
級数展開等
テイラー級数	taylor_series
一般化連分数	generalized_continued_fraction
フーリエ級数	fourier_series
変換
対応する変換	corresponding_transform
変換公式	corresponding_transform_formula
notes

数学的な関数の情報を集約するテンプレートです。

フォーマット

{{Infobox mathematical function
| name = 
| image= | imagesize= <!--(default 220px)--> | imagealt= | caption=
| generic_definition= | deriver= | motivation_of_creation= | date= | extends= | fields_of_application= | main_applications=
| domain= | codomain= | range=
| parity= | period= | analytic= | meromorphic= | holomorphic=
| zero= | plusinf= | minusinf= | max= | min= | vr1= | f1= | vr2= | f2= | vr3= | f3= | vr4= | f4= | vr5= | f5=
| asymptote= | root= | critical= | inflection= | fixed= | poles=
| reciprocal= | inverse= | derivative= | antiderivative= | other_related=
| taylor_series= | generalized_continued_fraction= | fourier_series=
| corresponding_transform= | corresponding_transform_formula=
| notes =
}}

使用例

正弦（Sine、サイン）関数
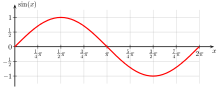基礎的な情報
一般の定義	$\sin(\alpha )={\frac {\text{opposite}}{\text{hypotenuse}}}$
考案者	ヒッパルコス
導入の動機	測量、天文学
考案時期	古代ギリシア
応用分野	三角法、積分変換、ほか多数
領域
定義域	(−∞, +∞) a
値域	[−1, 1] a
基本的な特徴
偶奇	奇関数
周期	2π
特筆すべき値
原点での値	0
極大値	2kπ + π/2 において 1 b
極小値	2kπ − π/2 において −1 b
その他の特徴
零点	kπ
臨界点	kπ + π/2
変曲点	kπ
不動点	0
関連する関数
逆数	Cosecant
逆関数	Arcsine
導関数	$f'(x)=\cos(x)$
原始関数	$\int f(x)\,dx=-\cos(x)+C$
その他	cos, tan, sec, cot
級数展開等
テイラー級数	$x-{\frac {x^{3}}{3!}}+{\frac {x^{5}}{5!}}-{\frac {x^{7}}{7!}}+\cdots =\sum _{n=0}^{\infty }{\frac {(-1)^{n}}{(2n+1)!}}x^{2n+1}$
一般化連分数	${\cfrac {x}{1+{\cfrac {x^{2}}{2\cdot 3-x^{2}+{\cfrac {2\cdot 3x^{2}}{4\cdot 5-x^{2}+{\cfrac {4\cdot 5x^{2}}{6\cdot 7-x^{2}+\ddots }}}}}}}}.$
a 実数について b k は 整数

{{Infobox mathematical function
| name = 正弦（Sine、サイン）関数
| image = Sine one period.svg

| general_definition = <math>\sin(\alpha) = \frac {\text{opposite}} {\text{hypotenuse}}</math>
| deriver=[[ヒッパルコス]]
| motivation_of_creation=[[測量]]、[[天文学]]
| date=[[古代ギリシア]]
| fields_of_application= [[三角法]]、[[積分変換]]、ほか多数
| domain=(−∞, +∞) {{smallsup|a}} |range=[−1, 1] {{smallsup|a}}
| parity=奇関数 | period=2{{pi}}
| zero=0 | max=2''k''{{pi}} + {{sfrac|{{pi}}|2}} において 1 {{smallsup|b}} |min=2''k''{{pi}} − {{sfrac|{{pi}}|2}} において −1 {{smallsup|b}}
| asymptote= |root=''k''{{pi}} |critical=''k''{{pi}} + {{sfrac|{{pi}}|2}} |inflection=''k''{{pi}} |fixed=0
| notes = {{ublist |{{sup|a}} [[実数]]について |{{sup|b}} ''k'' は [[整数]] }}

|reciprocal = [[Cosecant]]
|inverse = [[Arcsine]]
|derivative = <math>f'(x) = \cos(x) </math>
|antiderivative = <math>\int f(x)\,dx = -\cos(x) + C </math>
|generalized_continued_fraction = <math>
\cfrac{x}{1 + \cfrac{x^2}{2\cdot3-x^2 +
\cfrac{2\cdot3 x^2}{4\cdot5-x^2 +
\cfrac{4\cdot5 x^2}{6\cdot7-x^2 + \ddots}}}}.
</math>
|taylor_series= <math>
x - \frac{x^3}{3!} + \frac{x^5}{5!} - \frac{x^7}{7!} + \cdots = \sum_{n=0}^\infty \frac{(-1)^n}{(2n+1)!}x^{2n+1}
</math>

|other_related= cos, tan, sec, cot
}}

ガンマ（Gamma）関数
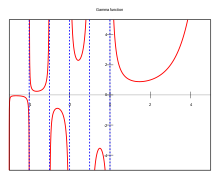実軸の一部におけるガンマ関数
基礎的な情報
一般の定義	$\Gamma (z)=\int _{0}^{\infty }x^{z-1}e^{-x}\,dx\$,$\qquad \Re (z)>0\$
考案者	レオンハルト・オイラー
導入の動機	階乗の 補間
考案時期	1729年
拡張元	階乗
応用分野	確率, 統計, 組合せ数学
主な応用先	ガンマ分布
領域
定義域	C ∖ Z≤ 0
値域	C ∖ {0}
基本的な特徴
偶奇	なし
周期	なし
解析的？	Yes
有理型？	Yes
正則？	Yes
特筆すべき値
極大値	なし
極小値	なし
n ∈ Z> 0での値	(n - 1) !
Z≤ 0での値	なし
その他の特徴
零点	なし
不動点	⊇ 1
極	Z≤ 0
変換
対応する変換	メリン変換
変換公式	$e^{-y}={\frac {1}{2\pi i}}\int _{c-i\infty }^{c+i\infty }\Gamma (s)y^{-s}\;ds$

{{Infobox mathematical function
| name = ガンマ（Gamma）関数
| image = Gamma plot.svg
| caption = 実軸の一部におけるガンマ関数

|general_definition = <math> \Gamma(z) = \int_0^\infty x^{z-1} e^{-x}\,dx \ </math>,<math>\qquad \Re(z) > 0\ </math>
| deriver = [[レオンハルト・オイラー]]
|motivation_of_creation = 階乗の [[補間]]
|extends = [[階乗]]
|date =[[1729年]]
|fields_of_application = [[確率]], [[統計]], [[組合せ数学]]
|main_applications = [[ガンマ分布]]

|domain={{math|'''C''' ∖ '''Z'''{{sub|≤ 0}}}} 
|range={{math|'''C''' ∖ {0}{{sup|}}}} 

|parity= なし
|period= なし
|analytic = Yes
|meromorphic = Yes
|holomorphic = Yes
|max= なし |min= なし |root= なし
|vr1={{math|''n'' ∈ '''Z'''{{sub|> 0}}}} |f1= {{math|(''n'' - 1) !}}
|vr2={{math|'''Z'''{{sub|≤ 0}}}} |f2= なし
|fixed={{math|⊇ 1}}
|poles = {{math|'''Z'''{{sub|≤ 0}}}} 

| corresponding_transform = [[メリン変換]]
| corresponding_transform_formula = <math>e^{-y}= \frac{1}{2\pi i} \int_{c-i\infty}^{c+i\infty} \Gamma(s) y^{-s}\;ds</math>
}}

追跡カテゴリ

• Category:不明な引数を指定しているページ/Template:Infobox mathematical function (0)

テンプレートデータ

TemplateData

これはビジュアルエディターやテンプレートウィザードにより使用されるテンプレートのためのTemplateData文書です。

Infobox mathematical function

数学的な関数の情報を集約するテンプレートです。

テンプレートパラメーター[テンプレートデータを編集]

パラメーター		説明	型	状態
name	name	関数名	内容	必須
image	image	画像ファイル名	内容	省略可能
imagesize	imagesize	画像サイズ	内容	省略可能
imagealt	imagealt	画像代替テキスト	内容	省略可能
caption	caption	画像のキャプション	内容	省略可能
generic_definition	generic_definition	関数の一般的な定義	内容	省略可能
deriver	deriver	考案・導入した人物	内容	省略可能
motivation_of_creation	motivation_of_creation	背景・動機	内容	省略可能
date	date	導入された時期	内容	省略可能
extends	extends	拡張元の関数	内容	省略可能
fields_of_application	fields_of_application	応用されている分野・領域	内容	省略可能
main_applications	main_applications	主な応用例	内容	省略可能
domain	domain	定義域	内容	省略可能
codomain	codomain	終域	内容	省略可能
range	range	値域	内容	省略可能
parity	parity	関数の偶奇性	内容	省略可能
period	period	関数の周期性	内容	省略可能
analytic	analytic	解析関数か否か	内容	省略可能
meromorphic	meromorphic	有理型関数か否か	内容	省略可能
holomorphic	holomorphic	正則関数か否か	内容	省略可能
zero	zero	原点での値	内容	省略可能
plusinf	plusinf	正の無限大における極限値	内容	省略可能
minusinf	minusinf	負の無限大における極限値	内容	省略可能
max	max	極大値	内容	省略可能
min	min	極小値	内容	省略可能
vr1	vr1	特筆すべき値をとる点1	内容	省略可能
f1	f1	vr1での値	内容	省略可能
vr2	vr2	特筆すべき値をとる点2	内容	省略可能
f2	f2	vr2での値	内容	省略可能
vr3	vr3	特筆すべき値をとる点3	内容	省略可能
f3	f3	vr3での値	内容	省略可能
vr4	vr4	特筆すべき値をとる点4	内容	省略可能
f4	f4	vr4での値	内容	省略可能
vr5	vr5	特筆すべき値をとる点5	内容	省略可能
f5	f5	vr5での値	内容	省略可能
asymptote	asymptote	漸近線	内容	省略可能
root	root	関数の零点	内容	省略可能
critical	critical	関数の臨界点	内容	省略可能
inflection	inflection	関数の変曲点	内容	省略可能
fixed	fixed	関数の不動点	内容	省略可能
poles	poles	関数の極	内容	省略可能
reciprocal	reciprocal	逆数	内容	省略可能
inverse	inverse	逆関数	内容	省略可能
derivative	derivative	導関数	内容	省略可能
antiderivative	antiderivative	原始関数	内容	省略可能
other_related	other_related	その他の関連のある関数	内容	省略可能
taylor_series	taylor_series	テイラー級数	内容	省略可能
generalized_continued_fraction	generalized_continued_fraction	一般化連分数	内容	省略可能
fourier_series	fourier_series	フーリエ級数	内容	省略可能
corresponding_transform	corresponding_transform	対応する変換操作	内容	省略可能
corresponding_transform_formula	corresponding_transform_formula	対応する変換操作の変換公式	内容	省略可能
notes	notes	注	内容	省略可能

関連項目

この解説は、Template:Infobox mathematical function/docから呼び出されています。 (編集 | 履歴)
編集者は、このテンプレートをサンドボックス (作成 | 複製)とテストケース (作成)で試すことができます。（解説）
/docのサブページにカテゴリを追加してください。 このテンプレートのサブページ一覧。